Concepts
- Whenever a number (x) is multiplied three times, then the resultant number is known as the cube of that number.
- To cube a number, just use it in a multiplication 3 times ...
-
The cube of a two digit number will clearly have less than seven digits. Eg. The highest two digit number is 99, whose cube is 993=970299, which is a six digit number.
- which of the following are cubes of even natural numbers?
216, 512, 729, 1000 , 3375 , 13824- We know that the cube of an even natural number is also an even a natural number
∴216 , 512,1000 , 13824 are even natural numbers. ∴ These can be the cube of even natural number.
- We know that the cube of an even natural number is also an even a natural number
-
-
- 0 cubed=03=0 × 0 × 0=0
- 1 cubed=13=1 × 1 × 1=1
- 2 cubed=23=2 × 2 × 2=8
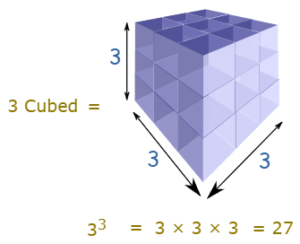
- 3 cubed=33=3 × 3 × 3=27
- 4 cubed=43=4 × 4 × 4=64
- 5 cubed=53=5 × 5 × 5=125
- 6 cubed=63=6 × 6 × 6=216
-
- 3 cubed is 27, so the cube root of 27 is 3
Example: What is the Cube root of 125?
Well, we just happen to know that 125 = 5 × 5 × 5 (if you use 5 three times in a multiplication you will get 125) ...
... so the cube root of 125 is 5
So the cube root of −125 is −5
The Perfect Cubes are the cubes of the whole numbers:
| Perfect Cubes |
|
| 0 | 0 |
| 1 | 1 |
| 2 | 8 |
| 3 | 27 |
| 4 | 64 |
| 5 | 125 |
| 6 | 216 |
| 7 | 343 |
| 8 | 512 |
| 9 | 729 |
| 10 | 1000 |
| 11 | 1331 |
| 12 | 1728 |
| 13 | 2197 |
| 14 | 2744 |
| 15 | 3375 |
It is easy to work out the cube root of a perfect cube, but it is really hard to work out other cube roots.
Example: what is the cube root of 30?
Well, 3 × 3 × 3 = 27 and 4 × 4 × 4 = 64, so we can guess the answer is between 3 and 4.
- Let's try 3.5: 3.5 × 3.5 × 3.5 = 42.875
- Let's try 3.2: 3.2 × 3.2 × 3.2 = 32.768
- Let's try 3.1: 3.1 × 3.1 × 3.1 = 29.791
We are getting closer, but very slowly ... at this point, I get out my calculator and it says:
3.1072325059538588668776624275224...
... but the digits just go on and on, without any pattern. So even the calculator's answer is only an approximation !
If n is the number, then cube of n is n × n × n (3 factors) and it is denoted by n cubed. Thus, the exponent of the cube of a number is 3.
| Number | Cube of the Number /Number Cubed |
| 1 | = 1 |
| 2 | =8 |
| 3 | = 27 |
| 4 | = 64 |
| 5 | =125 |
| 6 | = 216 |
| 7 | = 343 |
| 8 | = 512 |
| 9 | = 729 |
The above table gives the cubes of numbers from 1 to 9.
Pattern
Let us now understand the pattern of the units digits of the cubes of the numbers.
| Units digit of the number (n) | Units digit of the cube (n3n3) |
| 0, 1, 4, 5, 6, 9
|
0, 1, 4, 5, 6, 9
respectively |
| 2 | 8 |
| 3 | 7 |
| 7 | 3 |
| 8 | 2 |
Numbers with units digits 0, 1, 4, 5, 6, 9, the units digits of their cubes are again the same digits 0, 1, 4, 5, 6, 9.
Till now we have understood the idea of a cube and cube of a negative number and some other properties of cubes.
Observe below solution:
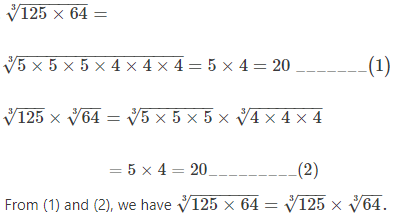


Questions & Answers
Which of the following numbers are perfect cubes? In case of perfect cube, find the number whose cube is the given number.
(i) 125 (ii) 243 (iii) 343 (iv) 256 (v) 8000 (vi) 9261 (vii) 5324 (viii) 3375
(i) 125=5×5×5= 53
By making them in group of 3 equal factor. We see no factor is left. ∴ It is a perfect cube.
(ii) 243=3×3×3 =33
By making them in group of 3 equal factor. We see no factor is left. ∴ It is a perfect cube.
Similarly we can solve others. Let see the example of non perfect cube:
(vii) 5324 = 2×2×11×11×11 =113
By making them in group of 3 equal factors, we see that 2×2 are left. ∴ It is not a perfect cube.
The square of a natural number subtracted from its cube is 48. the number is
Solution
In this case, the square of a number is subtracted from the cube of the number to give 48 as result.
Let us assume first that the number is to be X.
Then, Square of the natural number is x²
Again, cube of the same natural number is x³
According to the problem,
x³ - x² = 48
or, x²(x-1) = 48
or, x²(x-1) = 4²(4-1) as 4 raised to the power of 2 gives 16 and 4-1 is 3. (16 x 3=48)
This signifies that the value of X is 4 in this problem.
Ans. The value of X is 4 i.e. the natural number.

